Problem 9(a) of 2002 Euclid

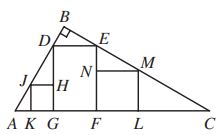
“In triangle ABC, \angle ABC = 90^{\circ}. Rectangle DEFG is inscribed in \Delta ABC, as shown. Squares JKGH and
MLFN are inscribed in \Delta AGD and \Delta CFE, respectively. If the side length of JHGK is v, the side length
of MLFN is w, and DG = u, prove that u =v+w.”

(The image is borrowed from the CEMC)

Notice that given a right triangle, the side length of the inscribed square as shown above can be expressed using two side lengths of the right triangle. Look at the following diagram.

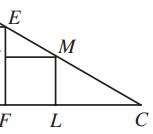
It’s easy to see ML:EF=CL:CF, then w:EF=(CF-w):CF.

Therefore we have w=\frac{EF*CF}{EF+CF}. This result is general. So, similarly, we can have the following equation immediately.

v=\frac{AG*DG}{AG+DG}

By \Delta AGD \sim \Delta EFC, we have \frac{DG}{AG}=\frac{CF}{EF}=r.

Therefore, AG=\frac{u}{r} and CF=ur. By substitutions, we have the following equation:

v+w=\frac{\frac{u}{r}u}{\frac{u}{r}+u}+\frac{u*ur}{u+ur} = u. Done.

Leave a Reply

Your email address will not be published. Required fields are marked *